RWA #1 Unit M Concept 5 elipses
1. Ellipse - The set of all points such that the sum of the distance from two points is a constant.
The ellipse
The ellipse
f
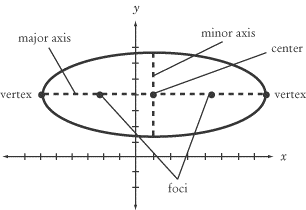
2. To find the ellipse it has to be in standard conic form which is (x-h)^2/a^2+ (y-k)^2/b^2 = 1. It doesn't matter in what order a^2 and b^2 are they can be under whichever equation. h and k have to be in a specific one. H always has to be with x and k always has to be with y. another thing is that to determine the size of the ellipse if it's skinny or fat has to be based on x or y. If there ia a bigger number under x then it's fat, but if it's under the y then it's skinny. As you can see on the picture whatever d and d 2 are when they are added they equal 2a.
When graphed h,k will be center points. What ever numbers are on the bottom like we said then it place will be a and the second one b.vertices come from a, co-vertices from b, and foci from c and we find c from a^2-b^2=c^2. If a has bigger numbers then it's our major axis and b will be minor axis. The foci will be on the major axis but a little before the vertices points. To determine the eccentricity of an ellipse,is e = c/a. So it's whatever number is c comes out to be divided by whatever number a is.
When graphed h,k will be center points. What ever numbers are on the bottom like we said then it place will be a and the second one b.vertices come from a, co-vertices from b, and foci from c and we find c from a^2-b^2=c^2. If a has bigger numbers then it's our major axis and b will be minor axis. The foci will be on the major axis but a little before the vertices points. To determine the eccentricity of an ellipse,is e = c/a. So it's whatever number is c comes out to be divided by whatever number a is.
3.A great example for and an ellipse would be a chain on a bicycle. As your feet move on the handle . The hardest part or the part where its the is the parking a lot. and the chain is the circle that keep revolving
4.
1 http://www.sparknotes.com/testprep/books/sat2/math2c/chapter8section4.rhtml
2 http://www.youtube.com/watch?v=-OPR7Krjj7c
3 http://mathforum.org/library/drmath/view/62576.html
2 http://www.youtube.com/watch?v=-OPR7Krjj7c
3 http://mathforum.org/library/drmath/view/62576.html
No comments:
Post a Comment